Home | Contact Us | About us | Mission Statement | Staff and Faculty | History of Our School | Admissions | Apply to SHS | Tuition and Fees | SHS Calendar | Education | Donate to SHS
Our mission
St. Herman of Alaska Christian School proves that education is more than a collection of facts or skills required to “make it” in this world, but it is the formation of the mind, body, and soul as a servant for our life with Christ.
SHS at a glance
-

25+ Years of Orthodox Christian Education
-
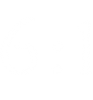
Student/Faculty Ratio 6:1
-

SHS serves all Orthodox jurisdictions and families from all ethnic backgrounds
-

SHS graduates accepted to Boston Latin School, Lexington Christian Academy, Boston Trinity Academy, B.C. High, Newman Prep
-

SHS offers a spiritually and academically rich and rigorous classical education
Saint Herman of Alaska
“Of all the features of the Elder’s face reflecting goodness; his pleasant smile, his meek and attractive mien, his humble and quiet behavior, and his gracious word. He was short of stature. His face was pale and covered with wrinkles. His eyes were greyish-blue, full of sparkle, and on his head there were a few gray hairs. His voice was not powerful, but it was very pleasant.” - Yanovsky
Learn More


